This page gives a brief overview of platforms and microresonator geometries that have been used in literature to generate Kerr frequency combs. A more general overview of microresonators (or microcavities) can also be found in a review article from K. Vahala from 20031. The references that are given here are supposed to just give an idea of the available platforms and not reference every work that has used these platforms. However, if you feel that there are important references missing, please let me know. The given quality factors are ranges typical for around 1550 nm, if not noted otherwise.
On-chip whispering-gallery mode resonators
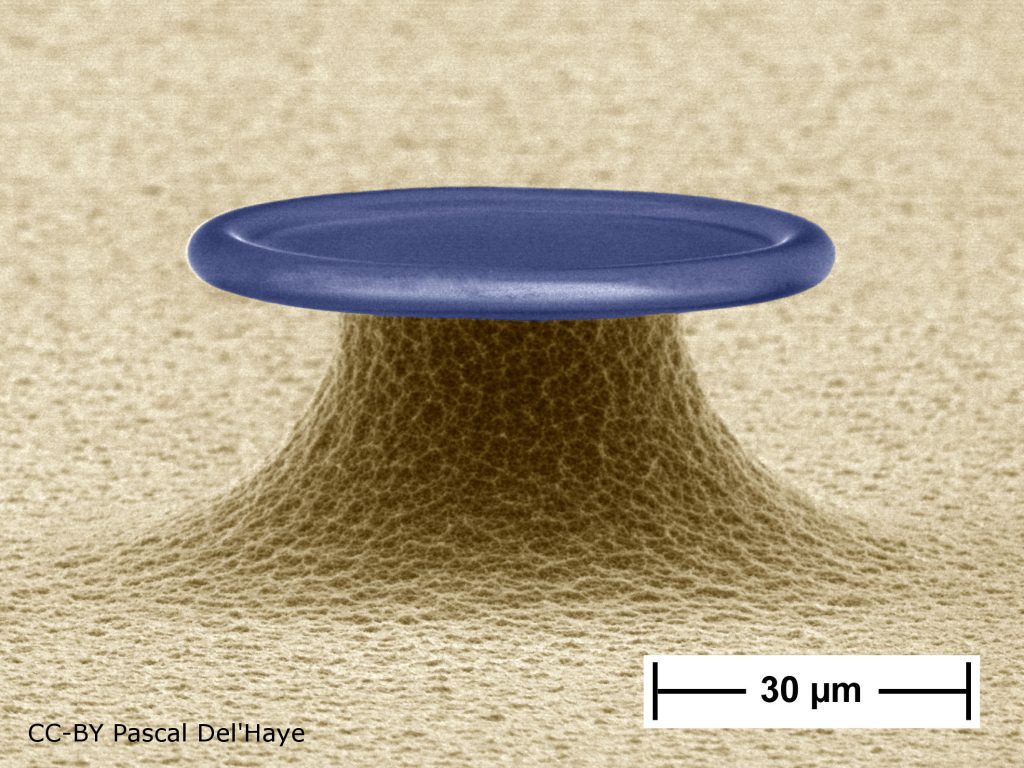
There are currently two variations of this whispering-gallery mode geometry in use:
- micro-toroids2
- micro-disks3
Both geometries share many features. The actual microresonator is of circular shape and in most cases made from silicon dioxide. The microresonator is suspended (see picture above) such that the cladding is typically air. To make the micro-disks, micro-fabrication processes are used on chip-scale or wafer-scale. Micro-toroids are made of micro-disks by laser reflow2. This creates the donut-shaped edge geometry and results in a very smooth sidewall of the microresonator which reduces optical scattering losses inside the micro-toroid. A micro-toroid was famously used in the original paper on Kerr frequency combs4. However, within the last few years the fabrication techniques of micro-disks have advanced substantially, such that also without a reflow very high Q factors can be achieved3. For both cases, micro-disks and micro-toroids, mostly tapered optical fibers are typically used to couple light to the microresonator. However, efforts are made to integrate waveguides for coupling40. One advantage of the micro-disks compared to the micro-toroids is the better control of the dispersion which can be quite precisely manipulated by using multi-step designs5. Currently, only few new work is done on Kerr frequency combs with micro-toroids, with the latest publications from the Tanabe group6 and the Xiao group38, while the group of K. Vahala very actively fabricates and uses micro-disk resonators7,8.
Silicon dioxide micro-toroids:
Typical quality factors: 108 to 109
Typical free spectral ranges: 90 to 900 GHz4,9
Pump wavelengths: 1550 nm
Resonator modes: many
Silicon dioxide micro-disks:
Typical quality factors: 108 to 109
Typical free spectral ranges: 3 to 300 GHz7
Pump wavelengths: 1550 nm, 1064 nm37, 800 nm37
Resonator modes: many
Bulk whispering gallery mode resonators
As the micro-disks and micro-toroids, these microresonators are true whispering gallery mode resonators. They consist of a bulk transparent material with a cylindrical shape and small, rotational symmetric protrusions on the outside in order to confine the optical mode along the axis of the cylinder. Several crystalline materials have been used in literature as well as fused glass:
- Magnesium fluoride (MgF2)10
- Calcium fluoride (CaF2)11
- Silicon dioxide (SiO2)12
- Barium fluoride (BaF2)13,14
Currently the most popular material for these type of microresonators is magnesium fluoride10,15,16 but Kerr frequency combs in such resonators were first demonstrated with calcium fluoride11. For high purity crystalline bulk materials the intrinsic optical losses can be very low. Combined with the low roughness of the carefully polished surface of the resonator, this allows for the highest quality factors of all listed microresonators. Values above 1010 have been reported17. The traditional fabrication techniques typically involve a diamond turning step to shape the protrusion and a polishing step to smoothen the surface and to reach the highest quality factors. Recent advances in diamond turning also allows to skip the polishing if the quality factors are not required to be above 108 and allow for small, well-defined protrusions to reduce the number of modes down to single mode operation16. The high purity and low absorption of the crystalline materials also allows for their use for mid-infrared wavelength13,14,18 however, recently it has been shown that multi-phonon absorption limits the quality factor for wavelengths further into the mid IR13,14. Notably, the very first dissipative Kerr solitons were first observed in a MgF2 microresonator19 at a wavelength of 1550 nm. Fused glass microresonators can be machined using a laser from a fused silica rod12,20. The advantage of this approach is that the fabrication is very quick and offers a reasonable control over the geometry. However, the quality factors are not as high as for the best crystalline materials. For this category of microresonators the cladding material is the surrounding atmosphere and typically air. To couple light to the microresonators either tapered optical fibers or prisms are used. While the company OEwaves offers commercial products based on crystalline microresonators and also does research with them, other research groups (Chembo, Kippenberg, Yu) use them as well. The fused silica variation is also used by different groups (Diddams/Papp, Del’Haye).
Magnesium fluoride
Typical quality factors: 108 to 5·109
Typical free spectral ranges: 10 to 100 GHz
Pump wavelengths: 1550 nm10, 2.5 µm18,
Resonator modes: many to single mode16
Calcium fluoride
Typical quality factors: 109 to 1010
Typical free spectral ranges: 10 to 100 GHz
Pump wavelengths: 1550 nm11, 800 nm21,
Resonator modes: many (potentially single mode possible, similar to MgF216)
Fused silicon dioxide (laser machined):
Typical quality factors: 108 to 109
Typical free spectral ranges: 10 to 400 GHz12
Pump wavelength: 1550 nm
Resonator modes: many
Waveguide resonators

One platform that is very promising in the context of integrated photonics are waveguide microresonators. While many of the resonators are ring-shaped (as in the picture shown above) in principle any closed waveguide shape works and in particular larger waveguide resonators are typically folded. The first Kerr frequency combs in integrated waveguide microresonators were generated in silicon nitride22 and silicon oxynitride/Hydex23 microresonators but by now the list of materials encompasses quite a few other materials as well:
- Silicon nitride (Si3N4 sometimes also just abbreviated as SiN)22
- Silicon oxynitride (Hydex)23
- Silicon24
- Aluminum nitride (AlN)25
- Aluminum gallium arsenide (AlGaAs)26
- Diamond27
- Gallium Phosphide (GaP)41
- Lithium Niobate (LN)43
- Silicon Carbide (SiC)44
One major advantage of the waveguide microresonators is that the coupling to the microresonator via a bus waveguide is rigidly integrated into the planar device and typically the resonator is embedded into a cladding material (mostly silicon dioxide). These two properties make the microresonators robust to external perturbation such as vibrations and the ambient conditions. Another advantage is the planar geometry which in principle allows for integration with other photonic circuits. Additionally, choosing the cross section of the waveguide allows to modify the microresonator properties such as dispersion28, mode area and number of guided modes29. In general, thanks to the use of modern microfabrication techniques such as electron beam lithography or high-resolution deep UV lithography and precise material growth and processing a very good control of the geometry of the order of some tens of nanometers can be achieved. Silicon nitride is currently the most commonly used material of the ones listed, at least for experiments around 1550 nm. A few major groups are working with the silicon (oxy)nitride platform (Lipson/Gaeta, Kippenberg, Morandotti, Moss, Weiner, Wong) and very broad, even octave-spanning, coherent Kerr frequency comb spectra have been reported30,31. There have been substantial efforts by these groups to reduce the optical losses inside the microresonator. For geometries with high confinement, typical values for the quality factor remain around 106 to 107 with some reports32 of values well above 108. Some of the other materials, in particular diamond, have been reported but the available literature remains rather sparse. Aluminium nitride has the advantage of possessing a second-order nonlinearity which allows for frequency doubling inside the microresonator or on the same chip33, there are also reports of the use of crystalline AlN in order to make lower loss microresonators39 which also allow for soliton generation42. Silicon has been used for some work in the mid-IR region24,34 where the nonlinear absorption of silicon is much weaker.
Silicon nitride/silicon oxynitride (Hydex)
Typical quality factors: 106 to 107
Typical free spectral ranges: 10 GHz to 1 THz
Pump wavelengths: 1550 nm, 1000 nm35, 1300 nm31
Resonator modes: few to single mode
Silicon
Typical quality factors: 105 to 106 (around 2.5 to 3 µm)
Typical free spectral ranges: 10 to 200 GHz
Pump wavelengths: 3 µm34, 2.5 µm24
Resonator modes: few to single mode
Aluminum nitride
Typical quality factors: ~5·105
Typical free spectral ranges: 200 to 500 GHz
Pump wavelengths: 1550 nm
Resonator modes: few to single mode
Diamond
Typical quality factors: ~106
Typical free spectral ranges: 900 GHz
Pump wavelengths: 1550 nm
Resonator modes: few to single mode
Fabry-Pérot resonators
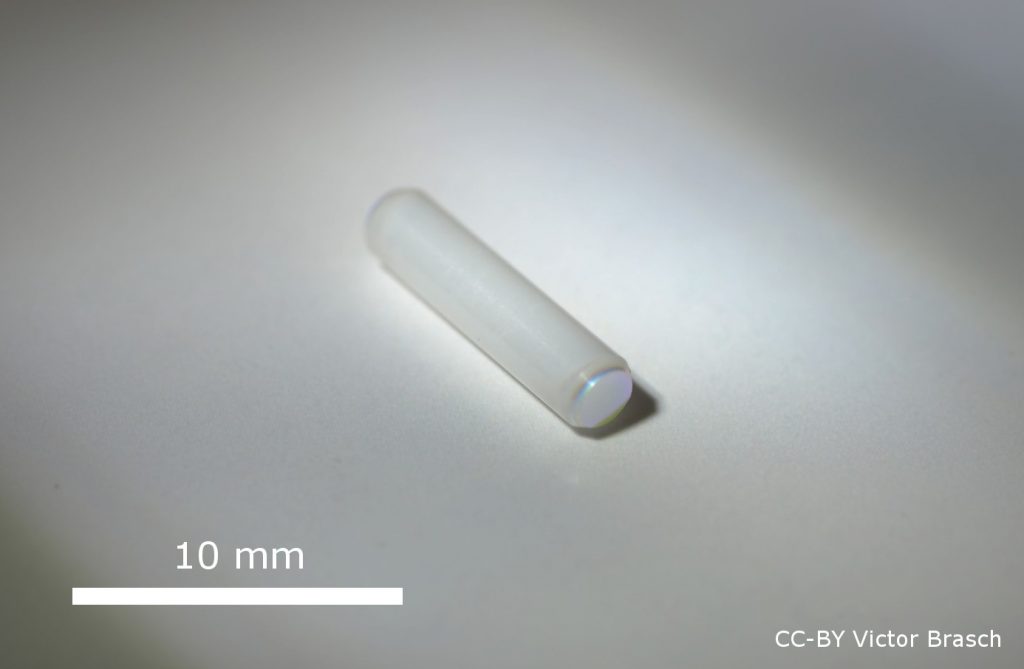
The most recent addition to the list of microresonator platforms used for Kerr frequency comb generation are Fabry-Pérot resonators36. Typical Fabry-Pérot resonators with a mirror on either side and air or vacuum in between, as they are often used as reference cavities, can not be used. They simply lack the nonlinear medium. However, when the air is replaced by a short piece of optical fiber this geometry can also be used to generate Kerr frequency combs and even dissipative soliton states36. This approach profits from the extremely low optical losses that are routinely achieved in modern optical fibers. The two parts of the microresonators, the mirrors and the fiber, also allow to adjust the microresonator properties. While it does not allow for the large design freedom of integrated microresonators it is in some sense more flexible than the whispering-gallery mode type of microresonators. One particular advantage of fiber based Fabry-Pérot microresonators is its intrinsic easy interfacing with the typical optical fiber environment which is used in most Kerr frequency comb laboratories and for optical data transmission.
Fiber-based Fabry-Pérot
Typical quality factors: 107 to 108
Typical free spectral ranges: 10 GHz
Pump wavelengths: 1550 nm
Resonator modes: single mode
Comparison for most common platforms
| SiO2 micro-disk | MgF2 crystalline | Si3N4 waveguide | |
| typical Q | 108 to 109 | 108 to 5·109 | 106 to 107 |
| FSR (GHz) | 3 to 300 | 10 to 100 | 10 to 1000 |
| pump λ (nm) | 800, 1060, 1550 | 1550, 2500 | 1060, 1550 |
| Robustness | – | –– | + |
| Potential for integration | o | – | ++ |
| Accessibility of resonator | + | ++ | – |
| Optical confinement | o | – | + |
| Optical nonlinearity | – | –– |
+ |
References
- Vahala, K. J. Optical microcavities. Nature 424, 839 (2003).
- Armani, D. K., Kippenberg, T. J., Spillane, S. M. & Vahala, K. J. Ultra-high-Q toroid microcavity on a chip. Nature 421, 925–928 (2003).
- Lee, H. et al. Chemically etched ultrahigh-Q wedge-resonator on a silicon chip. Nature Photonics 6, 369–373 (2012).
- Del’Haye, P. et al. Optical frequency comb generation from a monolithic microresonator. Nature 450, 1214–1217 (2007).
- Yang, K. Y. et al. Broadband dispersion-engineered microresonator on a chip. Nature Photonics 10, 316–320 (2016).
- Fujii, S., Kato, T., Suzuki, R. & Tanabe, T. Third-harmonic blue light generation from Kerr clustered combs and dispersive waves. Optics Letters 42, 2010 (2017).
- Yi, X., Yang, Q.-F., Yang, K. Y., Suh, M.-G. & Vahala, K. Soliton frequency comb at microwave rates in a high-Q silica microresonator. Optica 2, 1078 (2015).
- Yang, Q.-F., Yi, X., Yang, K. Y. & Vahala, K. Counter-propagating solitons in microresonators. Nat Photon 11, 560 (2017).
- Del’Haye, P. et al. Octave Spanning Tunable Frequency Comb from a Microresonator. Physical Review Letters 107, 063901 (2011).
- Liang, W. et al. Generation of near-infrared frequency combs from a MgF2 whispering gallery mode resonator. Opt. Lett., OL 36, 2290–2292 (2011).
- Savchenkov, A. A. et al. Tunable Optical Frequency Comb with a Crystalline Whispering Gallery Mode Resonator. Physical Review Letters 101, 093902 (2008).
- Del’Haye, P., Diddams, S. A. & Papp, S. B. Laser-machined ultra-high-Q microrod resonators for nonlinear optics. Applied Physics Letters 102, 221119 (2013).
- Grudinin, I. S., Mansour, K. & Yu, N. Properties of fluoride microresonators for mid-IR applications. Opt. Lett., OL 41, 2378–2381 (2016).
- Lecaplain, C., Javerzac-Galy, C., Gorodetsky, M. L. & Kippenberg, T. J. Mid-infrared ultra-high-Q resonators based on fluoride crystalline materials. Nature Communications 7, ncomms13383 (2016).
- Herr, T. et al. Universal formation dynamics and noise of Kerr-frequency combs in microresonators. Nature Photonics 6, 480–487 (2012).
- Grudinin, I. S. & Yu, N. Dispersion engineering of crystalline resonators via microstructuring. Optica, OPTICA 2, 221–224 (2015).
- Grudinin, I. S. et al. Ultra high Q crystalline microcavities. Optics Communications 265, 33–38 (2006).
- Wang, C. Y. et al. Mid-infrared optical frequency combs at 2.5 μm based on crystalline microresonators. Nature Communications 4, 1345 (2013).
- Herr, T. et al. Temporal solitons in optical microresonators. Nature Photonics 8, 145–152 (2013).
- Papp, S. B., Del’Haye, P. & Diddams, S. A. Mechanical Control of a Microrod-Resonator Optical Frequency Comb. Physical Review X 3, 031003 (2013).
- Savchenkov, A. A. et al. Kerr combs with selectable central frequency. Nature Photonics 5, 293–296 (2011).
- Levy, J. S. et al. CMOS-compatible multiple-wavelength oscillator for on-chip optical interconnects. Nature Photonics 4, 37–40 (2010).
- Razzari, L. et al. CMOS-compatible integrated optical hyper-parametric oscillator. Nature Photonics 4, 41–45 (2010).
- Griffith, A. G. et al. Silicon-chip mid-infrared frequency comb generation. Nature Communications 6, 6299 (2015).
- Jung, H., Xiong, C., Fong, K. Y., Zhang, X. & Tang, H. X. Optical frequency comb generation from aluminum nitride microring resonator. Optics Letters 38, 2810 (2013).
- Pu, M., Ottaviano, L., Semenova, E. & Yvind, K. Efficient frequency comb generation in AlGaAs-on-insulator. Optica, OPTICA 3, 823–826 (2016).
- Hausmann, B. J. M., Bulu, I., Venkataraman, V., Deotare, P. & Lončar, M. Diamond nonlinear photonics. Nature Photonics 8, 369–374 (2014).
- Riemensberger, J. et al. Dispersion engineering of thick high-Q silicon nitride ring-resonators via atomic layer deposition. Opt. Express, OE 20, 27661–27669 (2012).
- Kordts, A., Pfeiffer, M. H. P., Guo, H., Brasch, V. & Kippenberg, T. J. Higher order mode suppression in high-Q anomalous dispersion SiN microresonators for temporal dissipative Kerr soliton formation. Optics Letters 41, 452 (2016).
- Li, Q. et al. Stably accessing octave-spanning microresonator frequency combs in the soliton regime. Optica, OPTICA 4, 193–203 (2017).
- Pfeiffer, M. H. P. et al. Octave-spanning dissipative Kerr soliton frequency combs in Si3N4 microresonators. Optica 4, 684 (2017).
- Ji, X. et al. Ultra-low-loss on-chip resonators with sub-milliwatt parametric oscillation threshold. Optica 4, 619 (2017).
- Jung, H., Stoll, R., Guo, X., Fischer, D. & Tang, H. X. Green, red, and IR frequency comb line generation from single IR pump in AlN microring resonator. Optica, OPTICA 1, 396–399 (2014).
- Yu, M., Okawachi, Y., Griffith, A. G., Lipson, M. & Gaeta, A. L. Mode-locked mid-infrared frequency combs in a silicon microresonator. Optica 3, 854–860 (2016).
- Saha, K. et al. Broadband parametric frequency comb generation with a 1-μm pump source. Opt. Express, OE 20, 26935–26941 (2012).
- Obrzud, E., Lecomte, S. & Herr, T. Temporal solitons in microresonators driven by optical pulses. Nature Photonics 11, 600 (2017).
- Lee, S. H. et al. Towards visible soliton microcomb generation. Nature Communications 8, 1295 (2017).
- Ma, J., Jiang, X. & Xiao, M. Kerr frequency combs in large-size, ultra-high-Q toroid microcavities with low repetition rates [Invited]. Photon. Res., PRJ 5, B54–B58 (2017).
- Liu, X. et al. Integrated High-Q Crystalline AlN Microresonators for Broadband Kerr and Raman Frequency Combs. ACS Photonics (2018). doi:10.1021/acsphotonics.7b01254
- Yang, K. Y. et al. Bridging ultrahigh- Q devices and photonic circuits. Nature Photonics 12, 297–302 (2018).
- Wilson, D. J. et al. Integrated gallium phosphide nonlinear photonics. arXiv:1808.03554 [physics] (2018).
- Gong, Z. et al. High-fidelity cavity soliton generation in crystalline AlN micro-ring resonators. Opt. Lett., OL 43, 4366–4369 (2018).
- Wang, C., Zhang, M., Zhu, R., Hu, H. & Loncar, M. Monolithic photonic circuits for Kerr frequency comb generation, filtering and modulation. arXiv:1809.08637 [physics] (2018).
- Guidry, M. A. et al. Optical parametric oscillation in silicon carbide nanophotonics. Optica, OPTICA 7, 1139–1142 (2020).
